Exploring the Intersection: The Mathematics of Bell Ringing, Irish Music, and Scooby-Doo

What do chiming bells, Celtic music, and a cartoon about a talking dog and a group of meddling kids have in common? You probably wouldn’t say math, but Maisy Hoffman ’25 (she/her) would disagree. 

Alongside her mathematics and theatre double major, Hoffman moonlights as a WOZQ DJ, plays in the Wailing Banshees Celtic Music Ensemble, and is part of the Guild of Change Ringers on campus. 

Simply put, Hoffman sees math in everything, no matter the context. When dabbling in bell ringing, learning Wailing Banshee music, and watching her favorite TV show, she intuitively connects them to different math concepts.

As a member of the Guild of Change Ringers, Hoffman practices change ringing, a form of ringing tuned bells in controlled patterns which originated in the 17th century. She is currently pursuing a special studies at the intersection of math and change ringing, where she teaches herself the mathematical concept of group theory using her change ringing intuition. 

In change ringing, bells with various pitches are rung in scale from high to low. In each permutation of the pattern, a bell moves one position without repeating until the bells are back in scale. 

“[This creates] a really interesting mathematical problem of ‘how many patterns does this work for?’ For n bells, and as you get to more than five, or even just at five, it becomes a really, really high number. It gets gigantic,” Hoffman said.

As a result, change ringers created shorthands that condense and abstract patterns into commonalities. That’s where group theory comes into play. Hoffman explains that “all of those commonalities sort of founded group theory” even before mathematicians had formalized the concept and terminology. With this in mind, Hoffman decided to use her change ringing experience to dive into teaching herself group theory through special studies.

In addition to change ringing, as a member of the Wailing Banshees, Hoffman applies her mathematical perspective to Irish music. Irish music is played at a fast tempo and rarely with sheet music. As a result, Wailing Banshee members mostly learn music by ear.

“One of the things that I found really interesting about learning by ear is that there's so much subtle math involved,” Hoffman said.

Unlike other types of music, Irish music often strays from the typical 4/4 time signature, which is the number of beats in a musical bar. Irish music uses several other time signatures in styles like slide, jig, and slip jig tunes. At its core, time signatures boil down to math: counting and dividing beats in a measure of music.

Images Courtesy of Lily Serry

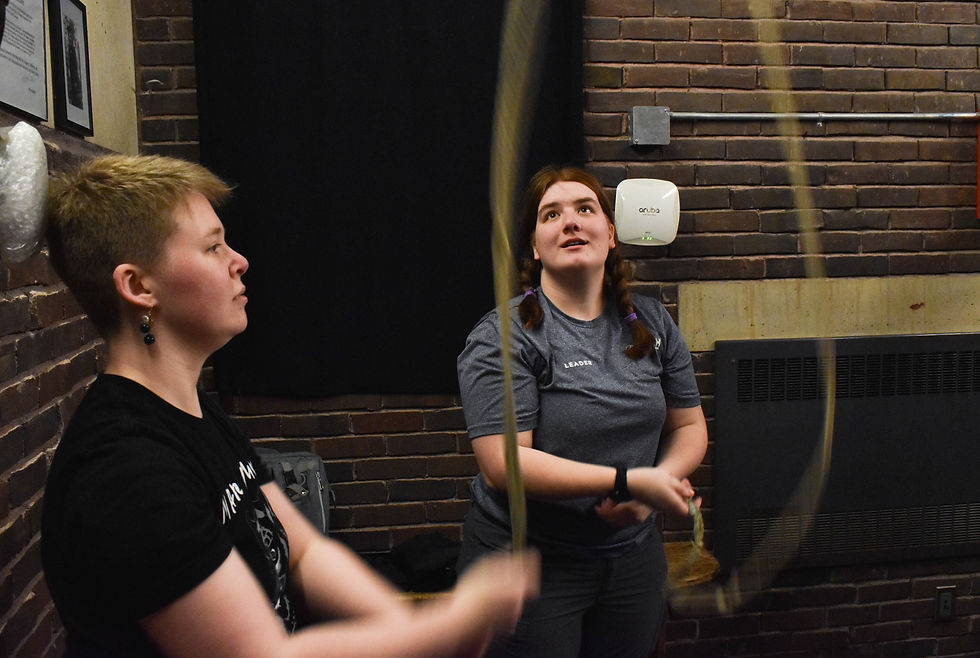
In her free time, Hoffman is also working on a passion project on her favorite TV show, Scooby-Doo. She and her sister, who have been watching the show since childhood, noticed that better episodes have many suspects and few culprits. This increases the probability of guessing the culprit’s identity wrong, adding mystery and anticipation. Hoffman hopes to create an equation to determine the best Scooby-Doo episode and started by looking at objective variables like suspect-to-culprit ratios.

However, Hoffman often finds subjectivity bleeding into objective variables. When does a character upgrade from a background character to a suspect? She has to define criteria to remain unbiased. But also, shouldn’t moments in the show that haven’t aged well—like racist scenes—lower the score? What if Hoffman thinks the plot is obvious or boring?

To account for the nuance, Hoffman decided to make two equations: an objective one focused only on the suspect-to-culprit ratio and a subjective one accounting for other judgment factors.

Hoffman is hoping to complete this project sometime next year. For now, she says a must-watch is A Scooby-Doo Halloween (What’s New Scooby-Doo? S2 E6) because it “has the best suspect-to-culprit ratio and is also so entertaining. It has a bunch of subtle, silly jokes, and the narrative is really fun.”

During her time at Smith, Hoffman has pursued her interests in art and math outside of class, and this has led to a seemingly unconventional combination of pursuits—group theory in change ringing, time signatures within Irish music, and equations in Scooby-Doo. As a senior, Hoffman urges students to take advantage of the extensive resources available to them beyond the classroom, whether that be special studies, extracurriculars, or passion projects.

“I like being in class, but I also love teaching myself,” Hoffman said. “I think especially with certain topics, it's so much more useful to teach yourself than to try to work with the curriculum that's already built.”

Written by Weina Lu, Lilly Butler, and Maggie Sullivan.